AP Physics 1 Simple Harmonic Motion MCQ: Embark on a journey to conquer the complexities of oscillatory motion. Delve into the equations that govern the dance of oscillating systems, explore the energy transformations that fuel their rhythm, and uncover the diverse applications that make SHM an indispensable tool across scientific disciplines.
Prepare to tackle the intricacies of displacement, velocity, and acceleration in SHM, unraveling the relationships that orchestrate their harmonious interplay. Discover the interplay of kinetic and potential energy, witnessing the seamless conversion that sustains the oscillations. Equip yourself with the problem-solving prowess to navigate the challenges posed by MCQs, unlocking the secrets of SHM through expert explanations.
1. Introduction: Ap Physics 1 Simple Harmonic Motion Mcq
Simple harmonic motion (SHM) is a periodic motion where an object oscillates around a central point with a constant amplitude. It occurs when a restoring force, proportional to the displacement from the equilibrium position, acts on the object.
Key characteristics of SHM include:
- Constant amplitude
- Periodic motion with a fixed frequency
- Restoring force proportional to displacement
- Zero net force at the equilibrium position
2. Equations of Motion

The equations of motion for SHM are:
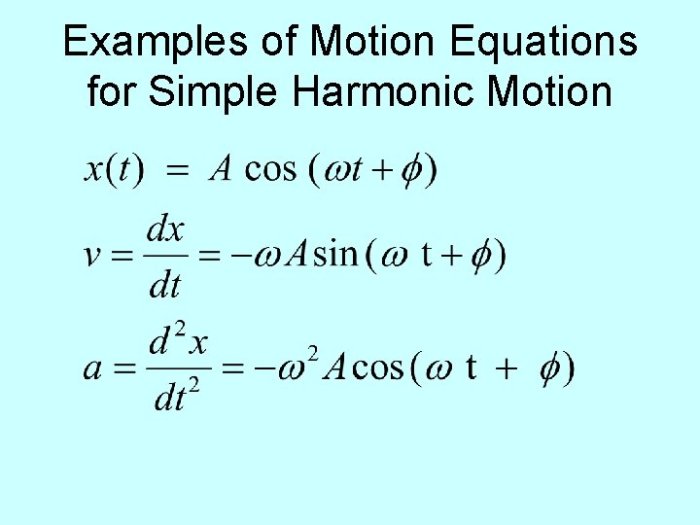
- Displacement: x = Acos(ωt + φ)
- Velocity: v =-Aωsin(ωt + φ)
- Acceleration: a =-Aω 2cos(ωt + φ)
where A is the amplitude, ω is the angular frequency, t is time, and φ is the phase angle.
These equations are related by the following:
v = dx/dt =
Aωsin(ωt + φ)
a = dv/dt =
Aω2cos(ωt + φ)
3. Energy in SHM
In SHM, energy is transferred between kinetic and potential energy. At the equilibrium position, all energy is potential energy. As the object moves away from equilibrium, potential energy is converted into kinetic energy. At the maximum displacement, all energy is kinetic energy.
The total energy of the system is given by:
E = 1/2kA2
where k is the spring constant.
4. Applications of SHM
SHM has numerous applications in various fields:
- Physics:Pendulums, springs, waves
- Engineering:Vibration analysis, resonance
- Biology:Oscillating molecules, heartbeat
- Music:Tuning forks, musical instruments
- Medicine:Ultrasound, MRI
User Queries
What is the equation for displacement in SHM?
x = A cos(ωt + φ)
How is energy transferred in SHM?
Energy is transferred between kinetic and potential energy as the system oscillates.
What are some real-world applications of SHM?
SHM is used in pendulums, springs, and vibrating strings, among other applications.